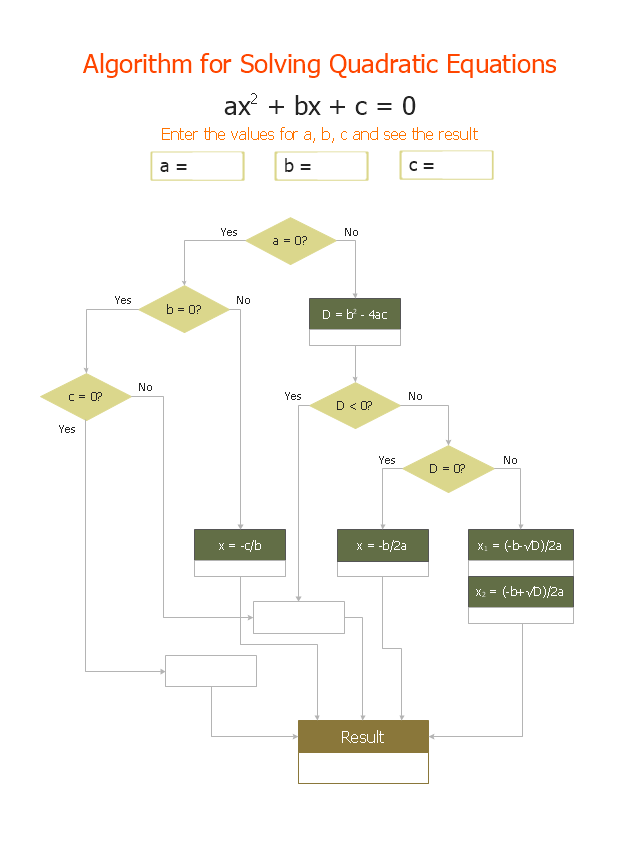
"In elementary algebra, a quadratic equation (from the Latin quadratus for "square") is any equation having the form
ax^2+bx+c=0
where x represents an unknown, and a, b, and c are constants with a not equal to 0. If a = 0, then the equation is linear, not quadratic. The constants a, b, and c are called, respectively, the quadratic coefficient, the linear coefficient and the constant or free term.
Because the quadratic equation involves only one unknown, it is called "univariate". The quadratic equation only contains powers of x that are non-negative integers, and therefore it is a polynomial equation, and in particular it is a second degree polynomial equation since the greatest power is two.
Quadratic equations can be solved by a process known in American English as factoring and in other varieties of English as factorising, by completing the square, by using the quadratic formula, or by graphing." [Quadratic equation. Wikipedia]
The flowchart example "Solving quadratic equation algorithm" was created using the ConceptDraw PRO diagramming and vector drawing software extended with the Mathematics solution from the Science and Education area of ConceptDraw Solution Park.
ax^2+bx+c=0
where x represents an unknown, and a, b, and c are constants with a not equal to 0. If a = 0, then the equation is linear, not quadratic. The constants a, b, and c are called, respectively, the quadratic coefficient, the linear coefficient and the constant or free term.
Because the quadratic equation involves only one unknown, it is called "univariate". The quadratic equation only contains powers of x that are non-negative integers, and therefore it is a polynomial equation, and in particular it is a second degree polynomial equation since the greatest power is two.
Quadratic equations can be solved by a process known in American English as factoring and in other varieties of English as factorising, by completing the square, by using the quadratic formula, or by graphing." [Quadratic equation. Wikipedia]
The flowchart example "Solving quadratic equation algorithm" was created using the ConceptDraw PRO diagramming and vector drawing software extended with the Mathematics solution from the Science and Education area of ConceptDraw Solution Park.
HelpDesk
How to Remove ConceptDraw Products from Mac and PC
CS Odessa provides registered users a 21 day trial period at no charge. A Trial License can be used to run a fully functional version of ConceptDraw products for 21 days. Trial mode functions exactly as a fully licensed copy of the product does. After your trial has expired and you have not yet purchased a license, the product reverts to Demo mode. The following article contains the instruction for those trial users who want to remove ConceptDraw products after the trial period is over.- Program And Flowchart For Root Of Quadratic Equation
- Flowchart Of Roots Of Quadratic Equation
- Flowchart To Find Roots Of Quadratic Equations
- Flow Chart Of The C Programme Solution Of A Quadratic Equation
- Quadratic Programming Flow Chart
- Flowchart To Find The Roots Of A Quadratic Equation
- Algorithm And Flowchart To Find Roots Of Quadratic Equation
- C Programming Examples With Flowchart
- Draw A Flowchart To Solve The Roots Of A Line Equation Of Degree 1
- Solving quadratic equation algorithm - Flowchart | Process ...
- Sample Project Flowchart . Flowchart Examples | Flowchart ...
- Process Flowchart | Solving quadratic equation algorithm ...
- C Programming Project Sample With Flowchart
- Basic Flowchart Symbols and Meaning | Process Flowchart | Good ...
- How to Create Flowchart Using Standard Flowchart Symbols | SDL ...
- Solving quadratic equation algorithm - Flowchart | Basic ...
- Basic Diagramming | Chemistry Equation Symbols | Chemistry ...
- Basic Flowchart Symbols and Meaning | Accounting Flowchart ...
- Euclidean algorithm - Flowchart | Solving quadratic equation ...
- Design elements - Involvement matrix | Customer types matrix ...